25 to 30% of the SAT math section will involve geometry, and the majority of those questions will deal with polygons in some form or another. Polygons come in many shapes and sizes and you will have to know your way around them with confidence in order to ace those SAT questions on test day.
Luckily, despite their variety, polygons are often less complex than they look, and a few simple rules and strategies will have you breezing through those geometry questions in no time.
This will be your complete guide to SAT polygons—the rules and formulas for various polygons, the kinds of questions you’ll be asked about them, and the best approach for solving these types of questions.
What is a Polygon?
Before we talk about polygon formulas, let’s look at what exactly a polygon is.
A polygon is any flat, enclosed shape that is made up of straight lines. To be “enclosed” means that the lines must all connect, and no side of the polygon can be curved.
Polygons

NOT Polygons

Polygons come in two broad categories—regular and irregular. A regular polygon has all equal sides and all equal angles, while irregular polygons do not.
Regular Polygons

Irregular Polygons

(Note: most all of the polygons on the SAT that are made up of five sides or more will be regular polygons, but always double-check this! You will be told in the question whether the shape is "regular" or "irregular.")
The different types of polygons are named after their number of sides and angles. A triangle is made of three sides and three angles (“tri” meaning three), a quadrilateral is made of four sides (“quad” meaning four), a pentagon is made of five sides (“penta” meaning five), and so on.
Most of the polygons you’ll see on the SAT (though not all) will either be triangles or some sort of quadrilateral. Triangles in all their forms are covered in our complete guide to SAT triangles, so let’s look at the various types of quadrilaterals you’ll see on the test.

With polygons, you may notice that many definitions will fit inside other definitions.
Quadrilaterals
There are many different types of quadrilaterals, most of which are subcategories of one another.
Parallelogram
A parallelogram is a quadrilateral in which each set of opposite sides is both parallel and congruent (equal) with one another. The length may be different than the width, but both widths will be equal and both lengths will be equal.

Parallelograms are peculiar in that their opposite angles will be equal and their adjacent angles will be supplementary (meaning any two adjacent angles will add up to 180 degrees).

Rectangle
A rectangle is a special kind of parallelogram in which each angle is 90 degrees. The rectangle’s length and width can either be equal or different from one another.

Square
If a rectangle has an equal length and width, it is called a square. This means that a square is a type of rectangle (which in turn is a type of parallelogram), but NOT all rectangles are squares.

Rhombus
A rhombus is a type of parallelogram in which all four sides are equal and the angles can be any measure (so long as their adjacents add up to 180 degrees and their opposite angles are equal). Just as a square is a type of rectangle, but not all rectangles are squares, a rhombus is a type of parallelogram (but not all parallelograms are rhombuses).

Trapezoid
A trapezoid is a quadrilateral that has only one set of parallel sides. The other two sides are non-parallel.

Kite
A kite is a quadrilateral that has two pairs of equal sides that meet one another.


And here come the formulas—mwahaha!
Polygon Formulas
Though there are many different types of polygons, their rules and formulas build off of a few simple basic ideas. Let’s go through the list.
Area Formulas
Most polygon questions on the SAT will ask you to find the area or the perimeter of a figure. These will be the most important area formulas for you to remember on the test.
Area of a Triangle
$$(1/2)bh$$
The area of a triangle will always be half the amount of the base times the height. In a right triangle, the height will be equal to one of the legs. In any other type of triangle, you must drop down your own height, perpendicular from the vertex of the triangle to the base.

Area of a Square
$$l^2 \or {lw}$$
Because each side of a square is equal, you can find the area by either multiplying the length times the width or simply by squaring one of the sides.
Area of a Rectangle
$$lw$$
For any rectangle that is not a square, you must always multiply the base times the height to find the area.
Area of a Parallelogram
$$bh$$
Finding the area of a parallelogram is exactly the same as finding the area of a rectangle. Because a parallelogram may slant to the side, we say we must use its base and its height (instead of its length and width), but the principle is the same.

You can see why the two actions are equal if you were to transform your parallelogram into a rectangle by dropping down straight heights and shifting the base.

Area of a Trapezoid
$$[(l_1+l_2)/2]h$$
In order to find the area of a trapezoid, you must find the average of the two parallel bases and multiply this by the height of the trapezoid.

Now let's look at an example:
In the figure, WXYZ is a rectangle with $\ov{WA} = \ov{BZ} = 4$. The area of the shaded region is 32. What is the length of $\ov{XY}$?

[Note: figure not to scale]
A. 6
B. 8
C. 12
D. 16
E. 20
First, let us fill in our given information.

Our shaded figure is a trapezoid, so let us use the formula for finding the area of a trapezoid.
area $=[(l_1+l_2)/2]h$
Now if we call the longest base q, the shortest base will be $q−4−4$, or $q−8$. (Why? Because the shortest leg is equal to the longest leg minus our two given lengths of 4).
This means we can now plug in our values for the leg lengths. In addition, we are also given a height and an area, so we can plug all of our values into the formula in order to find the length of our longest side, q.
$32=[(q+(q−8))/2]2$
$32=(2q+2q−16)/2$
$64=4q−16$
$80=4q$
$20=q$
The length of $\ov{XY}$ (which we designated $q$) is 20.
Our final answer is E, 20.
In general, the best way to find the area of different kinds of polygons is to transform the polygon into smaller and more manageable shapes. This will also help you if you forget your formulas come test day.
For example, if you forget the formula for the area of a trapezoid, turn your trapezoid into a rectangle and two triangles and find the area for each.
Let us look to how to solve the above problem using this method instead.

We are told that the area of the trapezoid is 32. We also know that we can find the area of a triangle by using the formula ${1/2}bh$. So let us find the areas for both our triangles.
${1/2}bh$
${1/2}(4)(2)$
${1/2}8$
$4$
Each triangle is worth 8, so together, both triangles will be:
$4+4$
$8$
Now if we add the area of our triangles to our given area of the trapezoid, we can see that the area of our full rectangle is:
$32+8$
$40$
Finally, we know that we find the area of a rectangle by multiplying the length times the width. We have a given width of 2, so the length will be:
$40=lw$
$40=2l$
20=l
The length of the rectangle (line $\ov{XY}$) will be 20.
Again, our final answer is E, 20.
Always remember that there are many different ways to find what you need, so don’t be afraid to use your shortcuts!
Angle Formulas
Whether your polygon is regular or irregular, the sum of its interior degrees will always follow the rules of that particular polygon. Every polygon has a different degree sum, but this sum will be consistent, no matter how irregular the polygon.
For example, the interior angles of a triangle will always equal 180 degrees (to see more on this, be sure to check out our guide to SAT triangles), whether the triangle is equilateral (a regular polygon), isosceles, acute, or obtuse.

All of these triangles will have a total interior degree measure of 180 degrees.
So by that same notion, the interior angles of a quadrilateral—whether kite, square, trapezoid, or other—will always add up to be 360 degrees. Why? Because a quadrilateral is made up of two triangles.

For example:
One interior angle of a parallelogram is 65 degrees. If the remaining angles have measures of $a$, $b$ and $c$, what is the value of $a+b+c$?
All quadrilaterals have an interior degree sum of 360, so:
$a+b+c+65=360$
$a+b+c=295$
The sum of $\bi a, \bi b$, and $\bi c$ is 295.
Interior Angle Sum
You will always be able to find the sum of a polygon’s interior angles in one of two ways—by memorizing the interior angle formula, or by dividing your polygon into a series of triangles.
Method 1: Interior Angle Formula
$$(n−2)180$$
If you have an $n$ number of sides in your polygon, you can always find the interior degree sum by the formula $(n−2)$ times 180 degrees.
If you picture starting from one angle and drawing connecting lines to every other angle to make triangles, you can see why this formula has an $n−2$. The reason being that you cannot make a triangle by using the immediate two connecting sides that make up the angle—each would simply be a straight line. To see this in action, let us look at our second method.
Method 2: Dividing Your Polygon Into Triangles
The reason the above formula works is because you are essentially dividing your polygon into a series of triangles. Because a triangle is always 180 degrees, you can multiply the number of triangles by 180 to find the interior degree sum of your polygon, whether your polygon is regular or irregular.

Individual Interior Angles
If your polygon is regular, you will also be able to find the individual degree measure of each interior angle by dividing the degree sum by the number of angles. (Note: $n$ can be used for both the number of sides and the number of angles; the number of sides and angles in a polygon will always be equal.)
$${(n−2)180}/n$$
Again, you can choose to either use the formula or the triangle dividing method by dividing your interior sum by the number of angles.

Angles, angler fish...same thing, right?
Side Formulas
As we saw earlier, a regular polygon will have all equal side lengths. And if your polygon is regular, you can find the number of sides by using the reverse of the formula for finding angle measures.
A regular polygon with n sides has equal angles of 120 degrees. How many sides does the figure have?
-
3
-
4
-
5
-
6
-
7
For this question, it will be quickest for us to use our answers and work backwards in order to find the number of sides in our polygon. (For more on how to use the plugging in answers technique, check out our guide to plugging in answers).
Let us start at the middle with answer choice C.
We know from our angle formula (or by making triangles out of our polygons) that a five sided figure will have:
$(n−2)180$
$(5−2)180$
$(3)180$
$540$ degrees.
Or again, you can always find your degree sum by making triangles out of your polygon.

This way you will still end up with $(3)180=540$ degrees.
Now, we also know that this is a regular polygon, so each interior angle will be this same. This means we can find the individual angles by dividing the total by the number of sides/angles.
So let us find the individual degree measures by dividing that sum by the number of angles.
$540/5=108$
Answer choice C was too small. And we also know that the more sides a figure has, the larger each individual angle will be. This means we can cross off answer choices A and B (60 degrees and 90 degrees, respectively), as those answers would be even smaller.
Now let us try answer choice D.
$(n−2)180$
$(6−2)180$
$(4)180$
$720$
Or you could find your internal degree sum by once again making triangles from your polygons.

Which would again give you $(4)180=720$ degrees.
Now let’s divide the degree sum by the number of sides.
$720/6=120$
We have found our answer. The figure has 6 sides.
Our final answer is D, 6.
 Luckily for us, the SAT is predictable. You don't need a psychic to figure out what you're likely to see come test day.
Luckily for us, the SAT is predictable. You don't need a psychic to figure out what you're likely to see come test day.
Typical Polygon Questions
Now that we’ve been through all of our polygon rules and formulas, let’s look at a few different types of polygon questions you’ll see on the SAT.
Almost all polygon questions will involve a diagram in some way (especially if the question involves any polygon with four or more sides). The few problems that do not use a diagram will generally be simple word problems involving rectangles.
Typically, you will be asked to find one of three things in a polygon question:
#1: The measure of an angle (or the sum of two or more angles)
#2: The perimeter of a figure
#3: The area of a figure
Let’s look at a few real SAT math examples of these different types of questions.
The Measure of an Angle:

Because this hexagon is regular, we can find the degree measure of each of its interior angles. We saw earlier that we can find this degree measure by either using our interior angle formula or by dividing our figure into triangles.
 A hexagon can be split into 4 triangles, so $180°*4=720$ degrees.
A hexagon can be split into 4 triangles, so $180°*4=720$ degrees.
There are 6 interior angles in a hexagon, and in a regular hexagon, these will all be equal. So:
$720/6=120$
Now the line BO is at the center of the figure, so it bisects the interior angle CBA. The angle CBA is 120, which means that angle $x$ will be:
$120/2=60$
Angle $x$ is 60 degrees.
Our final answer is B, 60.
The Perimeter of a Figure:

We are told that ABCE is a square with the area of 1. We know that we find the area of a square by multiplying the length and the width (or by squaring one side), which means that:
$lw=1$
This means that:
$l=1$
And,
$w=1$
We also know that every side is equal in a square. This means that $\ov{AB}, \ov{BC}, \ov{CE}, and \ov{AE}$ are ALL equal to 1.
We are also told that CED is an equilateral triangle, which means that each side length is equal. Since we know that $\ov{CE} = 1$, we know that $\ov{CD}$ and $\ov{DE}$ both equal 1 as well.
So the perimeter of the polygon as a whole—which is made of lines $\ov{AB}, \ov{BC}, \ov{CD}, \ov{DE}, and \ov{EA}$—is equal to:
$1+1+1+1+1=5$
Our final answer is B, 5.
[Note: don't get tricked into picking answer choice C! Even though each line in the figure is worth 1 and there are 6 lines, line $\ov{CE}$ is NOT part of the perimeter. This is an answer choice designed to bait you, so be careful to always answer only what the question asks.)
The Area of a Figure:

We are told that the length of the rug is 8 feet and that the length is also 2 feet more than the width. This means that the width must be:
$8−2=6$
Now we also know that we find the area of a rectangle by multiplying width and length. So:
$8*6=48$
The area of the rug is 48 square feet.
Our final answer is B, 48.
 And now time for some practical how-to's, from tying a bow to solving your polygon questions.
And now time for some practical how-to's, from tying a bow to solving your polygon questions.
How to Solve a Polygon Question
Now that we’ve seen the typical kinds of questions you’ll be asked on the SAT and gone through the process of finding our answers, we can see that each solving method has a few techniques in common.
In order to solve your polygon problems most accurately and efficiently, take note of these strategies:
#1: Break up figures into smaller shapes
Don’t be afraid to write all over your diagrams. Polygons are complicated figures, so always break them into small pieces when you can. Break them apart into triangles, squares, or rectangles and you’ll be able to solve questions that would be impossible to figure out otherwise.
Alternatively, you may need to expand your figures by providing extra lines and creating new shapes in which to break your figure. Just always remember to disregard these false lines when you’re finished with the problem.

Because this is an awkward shape, let us create a new line and break the figure into two triangles.

Next, let us replace our given information.

From our definitions, we know that every triangle will have interior angles that add up to 180 degrees. We also know that the two angles we created will be equal.

We can use this information to find the missing, equal, angle measures by subtracting our givens from 180 degrees.
$180−30−20−20$
$110$
Now, we can divide that number in half to find the measurement of each of the two equal angles.
$110/2$
$55$

Now, we can look at the smaller triangle as its own independent triangle in order to find the measure of angle z. Again, the interior angles will measure out to 180 degrees, so:
$180−55−55$
$70$
Angle $z$ is 70 degrees.
Our final answer is B, 70.
#2: Use your shortcuts
If you don’t feel comfortable memorizing formulas or if you are worried about getting them wrong on test day, don’t worry about it! Just understand your shortcuts (for example, remember that all polygons can be broken into triangles) and you’ll do just fine.
#3: When possible, use PIA or PIN
Because polygons involve a lot of data, it can be very easy to confuse your numbers or lose track of the path you need to go down to solve the problem. For this reason, it can often help you to use either the plugging in answer strategy (PIA) or the plugging in numbers strategy (PIN), even though it can sometimes take longer (for more on this, check out our guides to PIA and PIN).
#4: Keep your work organized
There is a lot of information to keep track of when working with polygons (especially once you break the figure into smaller shapes). It can be all too easy to lose your place or to mix-up your numbers, so be extra vigilant about your organization and don’t let yourself lose a well-earned point due to careless error.

Ready?
Test Your Knowledge
Now it's time to test your knowledge with real SAT math problems.
1.

2.

3.

Answers: D, B, 6.5
Answer Explanations
1. Again, when dealing with polygons, it's useful to break them into smaller pieces. For this trapezoid, let us break the figure into a rectangle and a triangle by dropping down a height at a 90 degree angle.
This will give us a rectangle, which means that we will be able to fill in the missing lengths.

Now, we can also find the final missing length for the leg of the triangle. Since this is a right triangle, we can use the Pythagorean theorem.
$a^2+b^2=c^2$
$x^2+15^2=17^2$
$x^2+225=289$
$x^2=64$
$x=8$

Finally, let us add up all the lines that make up the perimeter of the trapezoid.
$17+20+15+20+8$
$80$
Our final answer is D, 80.
2. We are told that the larger polygon has equal sides and equal angles. We can also see that the shaded figure has 4 sides and angles, which means it is a quadrilateral.
We know that a quadrilateral has 360 degrees, so let us subtract our givens from 360.
$x+y=80$
$360−80=280$
Again, we know that the polygon has all equal angles, so we can find the individual degree measures by dividing this found number in half.
$280/2=140$
Each interior angle of the polygon will have 140 degrees.
Now, we can find the number of sides by either reversing our polygon side formula or by plugging in answers. Let's look at both methods.
Method 1: Formula
$${(n−2)180}/n$$
We know that this formula gives us the measure of each interior angle, so let us use the knowledge of our individual interior angle (our found 140 degrees) and plug it in to find n, the number of sides.
$140={(n−2)180}/n$
$140n=(n−2)180$
$140n=180n−360$
$−40n=−360$
$n=9$
Our polygon has 9 sides.
Our answer is B, 9.
Method 2: Plugging in answers
We can also use our method of plugging in answers to find the number of sides in our polygon.
As always, let us select answer option C.
Answer choice C gives us 8 sides. We know that a polygon with eight sides will be broken into 6 triangles.

So it will have:
$180*6$
$1080$ degrees total
Now, if we divide this total by the number of sides, we get:
$1080/8$
$135$
Each interior angle will be 135 degrees.
This answer is close, but not quite what we want. We also know that the more sides a regular polygon has, the larger each interior angle measure will be (an equilateral triangle's angles are each 60 degrees, a rectangle's angles are each 90 degrees, and so on), so we need to pick a polygon with more than 8 sides.
Let us then try answer choice B, 9 sides.
We know that a 9-sided polygon will be made from 7 triangles.

This means that the total interior degree measure will be:
$180*7$
$1260$
And we know that each angle measure will be equal, so:
$1260/9$
$140$
We have found our correct answer—a 9-sided polygon will have individual angle measures of 140 degrees.
Our final answer is B, nine.
3. Let us begin by breaking up our figure into smaller, more manageable polygons.
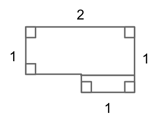
We know that the larger rectangle will have an area of:
$2*1$
$2$
The smaller rectangle will have an area of:

$1*x$
$x$
(Note: we are using $x$ in place of one of the smaller sides of the small rectangles, since we do not yet know its length)
We are told that the total area is $9/4$, so:
$2+x=9/4$
$x=9/4−2$
$x=9/4−8/4$
$x=1/4$
Now that we know the length of x, we can find the perimeter of the whole figure.

Let us add all of the lengths of our exposed sides to find our perimeter.
$1+2+1+0.25+1+0.25+1$
$6.5$
Our perimeter is $6.5.$
Our final answer is 6.5.

I think you deserve a present for pushing through on polygons, don't you?
The Take Aways
Though polygon questions may seem complicated, all polygons follow just a handful of rules. You may come across irregular polygons and ones with many sides, but the basic strategies and formulas will apply regardless.
So long as you follow your solve steps, keep your work well organized, and remember your key definitions, you will be able to take on and solve polygon questions that once seemed utterly obscure.
What’s Next?
Phew! You knocked out polygons and now it's time to make sure the rest of your math know-how is in top shape. First, make sure you have working knowledge of all the math topics on the SAT so that you can get a sense of your strengths and weaknesses. Next, find more topic-specific SAT math guides like this one so that you can turn those weak areas into strengths.
Need to brush up on your probability questions? Fractions and ratios? Lines and angles? No matter what topic you need, we've got you covered.
Running out of time on the SAT math? Look to our guide on how to best boost your time (and your score!).
Worried about test day? Take a look at how you should prepare for the actual day in question.
Want to get a perfect score? Check out our guide to getting an 800 on SAT math, written by a perfect scorer.